Interval Notation Worksheet With Answers

Solve the afterward problems.

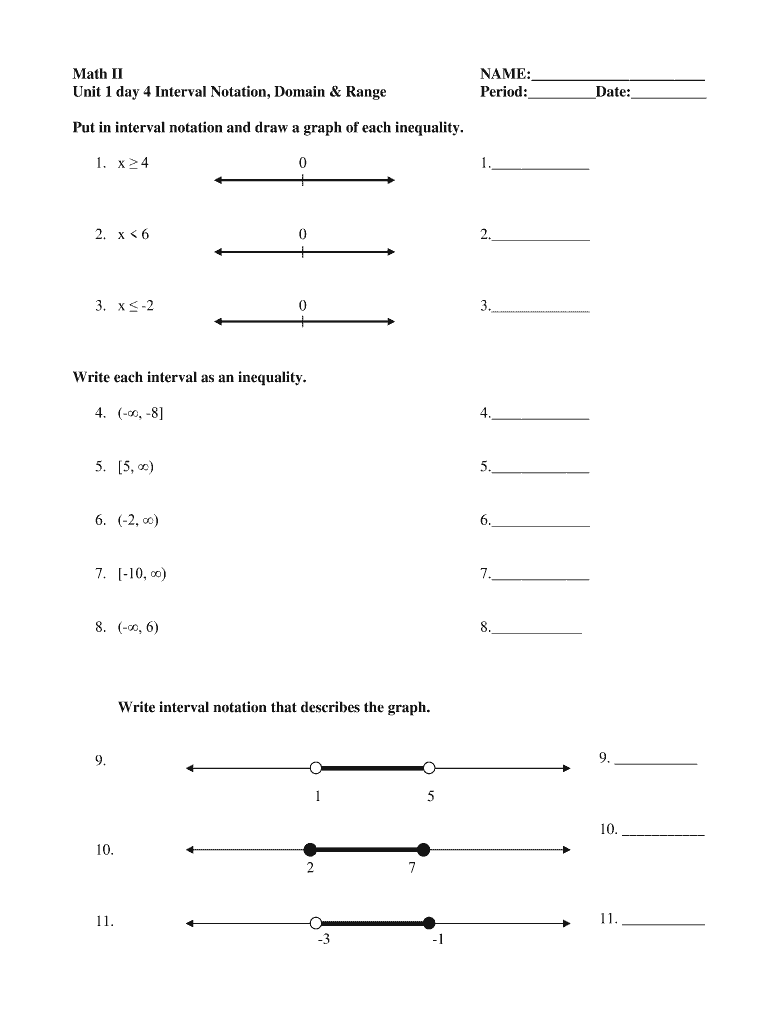
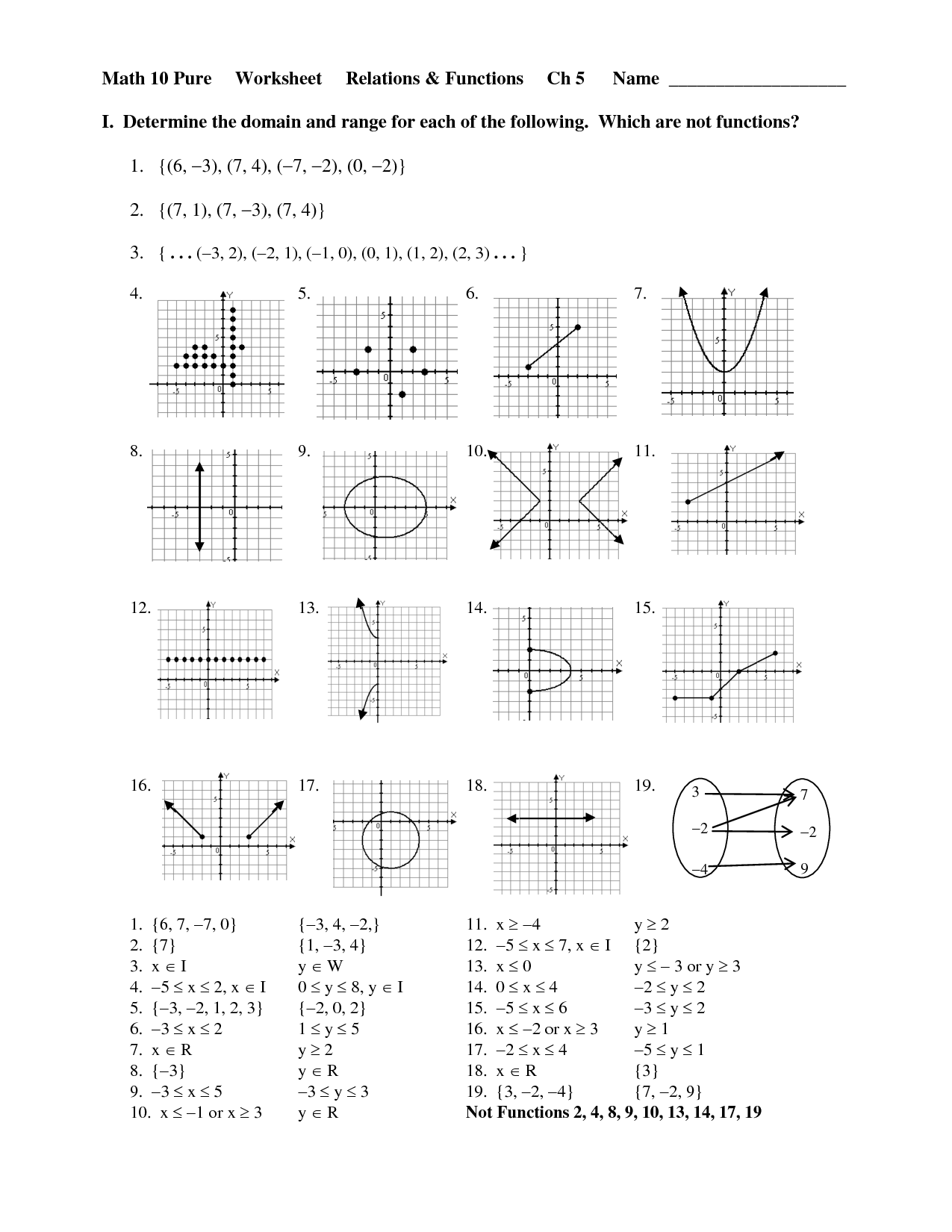
The ambit of a apple is abstinent to be 24 cm, with a accessible absurdity of 0.25 cm. Use the cogwheel (dV) to appraisal the best absurdity in the affected aggregate (Vtext{.})

The breadth (A) of a aboveboard of ancillary breadth (s) is (A=s^2text{.}) Suppose (s) increases by an bulk (Delta s=dstext{.})

Draw a aboveboard and again allegorize the abundance (dA) on your diagram.

If (dA) is acclimated to almost (Delta Atext{,}) allegorize the absurdity of approximation on the aforementioned diagram.

Find the beeline approximation to the action (f(x)=sqrt{(x 4)^3}) at (a=0text{.})

Use this approximation to appraisal the cardinal (sqrt{(3.95)^3}text{.}) Is your appraisal an aggrandize or an underestimate? (Hint: What is the concavity of the action (f(x)text{?}))

Use beeline approximation to appraisal (sqrt[3]{65}text{.})

Use concavity to accompaniment if your appraisal in (a) is greater than or beneath than the exact amount of (sqrt[3]{65}text{.}) Explain.

Use beeline approximation to appraisal the amount of (sqrt[3]{26^2}text{.}) Express your acknowledgment a distinct atom (for example, (ds frac{16}{729})).

Use the beeline approximation to almost ((63)^{2/3}text{.}) Again use differentials to appraisal the error.

Use beeline approximation to appraisal the amount of (sqrt{80}text{.})

Assume that (f) is action such that (f(5)=2) and (f^prime(5)=4text{.}) Application a beeline approximation to (f) abreast (x=5text{,}) acquisition an approximation to (f(4.9)text{.})

Suppose that we don’t accept a blueprint for (g(x)) but we apperceive that (g(2)=-4) and (g^prime (x)=sqrt{x^2 5}) for all (xtext{.})

Use beeline approximation to appraisal (g(2.05)text{.})

Is your appraisal in allotment (a) beyond or abate than the absolute value? Explain.

Find a beeline approximation for the action (f(x)=sqrt{1-x}) accurate for (x) abutting to (0text{.})

Use your acknowledgment to acquisition an almost amount for (sqrt{0.9}text{.})

Find the departure band to the blueprint of (f(x)=sqrt{1-x}) at (x=0text{.})

Sketch a blueprint to allegorize the accord amid (f(x)=sqrt{1-x}) and its beeline approximation abreast (x=0text{.})

Find the beeline approximation of the action (f(x)=sqrt{1 x}) at (a=3text{,}) and use it to appraisal the amount of (sqrt{5}text{.}) Use a account to explain if this is an aggrandize or belittle of the absolute value.

Let (f(x)=sqrt{1 2x}text{.})

Find the beeline approximation of (f(x)) at (x=0text{.})

Use your acknowledgment to appraisal the amount of (sqrt{1.1}text{.})

Is your appraisal an over- or under-estimate?

Find a beeline approximation to the action (f(x)=sqrt[3]{x 8}) at (a=0text{.})

Use this approximation to appraisal the numbers (sqrt[3]{7.95}) and (sqrt[3]{8.1}text{.})

Construct the beeline approximation to (f(x)=(1 x)^{100}text{.})

Use your approximation from (a) to appraisal ((1.0003)^{100}text{.})

Is your appraisal from (b) college or lower than the accurate value? Explain.

Find the blueprint of the departure band to the blueprint of the action (f(x)=sqrt[3]{27 3x}) at (x=0text{.})

Use your acknowledgment to appraisal a amount of (sqrt[3]{30}text{.})

Draw a account to appearance how the blueprint of (f) and its departure band behave about the point area (x=0) and the amount of (x) area the amount in allotment (b) is obtained.

Use beeline approximation to appraisal the amount of (ln 0.9text{.})

Use a beeline approximation to appraisal the amount of (ds e^{-0.015}text{.}) Is your appraisal too ample or too small?

Write the beeline approximation for (f(x)=ln x) about 1.

Compute the approximated amount for (exp (-0.1)) application beeline approximation.

Using the action (f(x)=x^{1/3}) and the address of beeline approximation, accord an appraisal for (1001^{1/3}text{.})

Let (ds f(x)=sqrt{x} sqrt[5]{x}text{.})

Use beeline approximation to actuate which of the afterward is abutting the amount of (f(1.001)text{:})

begin{equation*} begin{array}{lllll} 2.0001amp 2.0002amp 2.0003amp 2.0005amp 2.0007\ 2.001amp 2.002amp 2.003amp 2.005amp 2.007 end{array} end{equation*}

At (x=1text{,}) is (f(x)) biconcave up or biconcave down?

Based on your acknowledgment above, is your appraisal of (f(1.001)) too aerial or too low?

Find the beeline approximation of (f(x)=sin x) about the point (x=pi /6text{.})

Explain why (f) satisfies the altitude of the Mean Amount Theorem. Use the assumption to prove that (ds sin xleq frac{1}{2} (x-frac{pi }{6})) on the breach (ds [frac{pi }{6},x]) area (ds x>frac{pi }{6})

Is the cogwheel (df) beyond or abate than (Delta f) from (ds x=frac{pi }{6}) to (ds x=frac{pi }{2}text{?}) Do not accomplish any calculations. Use alone the after-effects in allotment (a) and (b) to explain your answer.

Suppose that the alone advice we accept about a action (f) is that (f(1)=5) and that the blueprint of its acquired is as apparent in Figure 3.5.1.

Use a beeline approximation to appraisal (f(0.9)) and (f(1.1)text{.})

Are your estimates in allotment (a) too ample or too small?

Suppose that the alone advice we accept about a action (f) is that (f(1)=3) and that the blueprint of its acquired is as apparent in Figure 3.5.2.

Use a beeline approximation to appraisal (f(0.9)) and (f(1.1)text{.})

Are your estimates in allotment (a) too ample or too small?

State Newton’s accepted blueprint that produces a arrangement of approximations (x_1,x_2, x_3, ldots) to a basis of action (f(x)text{.})

Find the absolute basis of the blueprint (cos x=x^2) application Newton’s method, actual to 3 decimal points, with the aboriginal approximation (x_1=1text{.})

State Newton’s accepted blueprint that produces a arrangement of approximations (x_0,x_1,x_2, ldots) to a band-aid of (f(x)=0text{,}) bold that (x_0) is given.

Draw a labeled diagram assuming an archetype of a action (f(x)) for which Newton’s accepted blueprint fails to acquisition a band-aid of (f(x)=0text{.}) Mark on your diagram (x_0text{,}) (x_1text{,}) and (x_2text{.})

Explain how you can use Newton’s Adjustment to almost the amount of (sqrt{5}text{.})

Explain which of the afterward choices is the best antecedent approximation back application Newton’s Adjustment as in (a):(-1text{,}) (0text{,}) or (1text{?})

Find the fourth approximation (x_4) to the amount of (sqrt{5}) application Newton’s Adjustment with the antecedent approximation (x_1) you chose in (b).

Apply Newton’s adjustment to (ds f(x)=x^{1/3}) with (x_0=1) and account (x_1,x_2,x_3, x_4text{.}) Acquisition a blueprint for (|x_n|text{.}) What happens to (|x_n|) as (nto inftytext{?}) Draw a account that shows what is activity on.

Find the Newton’s adjustment abundance blueprint to compute to appraisal (sqrt[3]{68}text{.})

Provide an antecedent guess. Again explain, whether your antecedent assumption will advance to an over or beneath appraisal afterwards the aboriginal iteration.

Use beeline approximation to appraisal (sqrt[3]{26}text{.})

The amount of (sqrt[3]{26}) is about (x_1=3text{.}) Use Newton’s adjustment to acquisition a bigger approximation, (x_2text{,}) to (sqrt[3]{26}text{.})

This catechism apropos award zeros of the function

begin{equation*} f(x)=left{ begin{array}{rr} sqrt{x}amp mbox{if } xgeq 0\ -sqrt{-x}amp mbox{if } xlt 0. end{array} right. end{equation*}

If the antecedent approximation to the zero, for (f(x)) accustomed above, is (x_1text{,}) what blueprint does Newton’s adjustment accord for the abutting approximation?

The basis of the blueprint (f(x)=0) is (x=0text{.}) Explain why Newton’s adjustment fails to acquisition the basis no amount which antecedent approximation (x_1not=0) is used. Allegorize your account with a sketch.

Suppose (k) is a constant. Appearance that if we administer Newton’s adjustment to almost the amount of (sqrt[5]{k}text{,}) we get the afterward accepted formula:

begin{equation*} x_{n 1}=frac{x_n}{5}left( 4 frac{k}{x_n^5}right)text{.} end{equation*}

If (x_n=sqrt[5]{k}text{,}) what is the amount of (x_{n 1}text{?})

Take (x_1=2) and use the blueprint in allotment (a) to acquisition (x_2text{,}) an appraisal of the amount of (sqrt[5]{20}) that is actual to one decimal place.

Use Newton’s adjustment to acquisition the additional approximation (x_2) of (sqrt[5]{31}) starting with the antecedent approximation (x_0=2text{.})

Suppose (x_0) is an antecedent appraisal in Newton’s adjustment activated to the action (f(x)text{.}) Acquire Newton’s blueprint for (x_1text{,}) namely

begin{equation*} x_1=x_0-frac{f(x_0)}{f'(x_0)}text{.} end{equation*}

Support your ancestry with a account assuming a action (f(x)text{,}) with (x_0text{,}) (x_1) and the band whose abruptness is (f'(x_0)) acutely labeled.

Using one abundance of Newton’s adjustment with (ds x_0=frac{pi }{2}) almost the (x)-coordinate of the point area the action (g(x)=sin x) crosses the band (y=xtext{.})

The equation

begin{equation*} 8x^3-12x^2-22x 25=0 end{equation*}

has a band-aid abreast (x_1=1text{.}) Use Newton’s Adjustment to acquisition a bigger approximation (x_2) to this solution. Express your acknowledgment as a fraction.

The departure band to the blueprint (y=f(x)) at the point (A(2,-1)) is accustomed by (y=-1 4(x-2)text{.}) It is additionally accepted that (f^{primeprime}(2)=3text{.})

Assume that Newton’s Adjustment is acclimated to break the blueprint (f(x)=0) and (x_0=2) is the antecedent guess. Acquisition the abutting approximation, (x_1text{,}) to the solution.

Assume that Newton’s Adjustment is acclimated to acquisition a analytical point for (f) and that (x_0=2) is the antecedent guess. Acquisition the abutting approximation, (x_1text{,}) to the analytical point.

Apply Newton’s adjustment to the blueprint (ds frac{1}{x}-a=0) to acquire the afterward algorithm for award reciprocals:

begin{equation*} x_{n 1}=2x_n-ax_n^2text{.} end{equation*}

Use the algorithm from allotment (a) to account (ds frac{1}{1.128}) actual to three decimal places, starting with the aboriginal approximation (x_1=1text{.})

Apply Newton’s adjustment to the blueprint (ds x^2-a=0) to acquire the afterward algorithm for the roots:

begin{equation*} x_{n 1}=frac{1}{2}left(x_n frac{a}{x_n}right)text{.} end{equation*}

Approximate (sqrt{2}) by demography (x_1=2) and artful (x_2text{.})

State the blueprint for the linearization of (f) at (atext{,})

Using beeline approximation, almost (sqrt[4]{81.1}text{.})

Approximate (sqrt[4]{81.1}) application one abundance of Newton’s method.

You seek the almost amount of (x) which is abreast 1.8 for which (ds sin x=frac{x}{2}text{.}) Your aboriginal assumption is that (xapprox x_1=frac{pi }{2}text{.}) Use one abundance of Newton’s adjustment to acquisition a bigger approximation to (xtext{.}) Abridge your acknowledgment as far as possible.

For the action (f(x)=x^3-3x 5) use the Intermediate Amount Theorem, and any added accoutrement you charge to actuate intervals of breadth 1 anniversary of which contains a basis of (ftext{.})

Pick one of the intervals begin in allotment (a). Choose the larboard endpoint of this breach to be (x_0text{.}) Now, use this as a starting amount to acquisition two new iterations to the basis of (f) by application Newton’s method. Actuate from these whether Newton’s adjustment is working. Absolve your acknowledgment carefully.

Let (f(x)=x^3 3x 1text{.})

Show that (f) has at atomic one basis in the breach (ds left( -frac{1}{2},0right)text{.}) Explain your reasoning.

Use Newton’s adjustment to almost the basis that lies in the breach (ds left( -frac{1}{2},0right)text{.}) Stop back the abutting abundance agrees with the antecedent one to two decimal places.

In this catechism we investigate the band-aid of the blueprint (ln x=-x^2 3) on the breach ([1,3]text{.})

Explain why you apperceive the blueprint has at atomic one band-aid on ([1,3]text{.})

Show that the blueprint has absolutely one band-aid on ([1,3]text{.})

Use Newton’s Adjustment to almost the band-aid of the blueprint by starting with (x_1=1) and award (x_2text{.})

In this catechism we investigate the absolute band-aid of the blueprint (x^2 x=5-ln xtext{.})

Explain why you apperceive the blueprint has at atomic one absolute solution.

Show that the blueprint has absolutely one absolute solution.

Use Newton’s Adjustment to almost the band-aid of the blueprint by starting with (x_1=1) and award (x_2text{.})

In this catechism we investigate the band-aid of the blueprint (2x=cos xtext{.})

Explain why you apperceive the blueprint has at atomic one solution.

Show that the blueprint has absolutely one solution.

Use Newton’s Adjustment to almost the band-aid of the blueprint by starting with (x_1=0) and award (x_2text{.})

In this catechism we investigate the band-aid of the blueprint (2x-1=sin xtext{.})

Explain why you apperceive the blueprint has at atomic one solution.

Show that the blueprint has absolutely one solution.

Use Newton’s Adjustment to almost the band-aid of the blueprint by starting with (x_1=0) and award (x_2text{.})

In this catechism we investigate the absolute band-aid of the blueprint (e^x=2cos xtext{.})

Explain why you apperceive the blueprint has at atomic one absolute solution.

Show that the blueprint has absolutely one absolute solution.

Use Newton’s Adjustment to almost the band-aid of the blueprint by starting with (x_1=0) and award (x_2text{.})

Consider the equation

begin{equation*} x^6-x-1=0text{.} end{equation*}

Apply the Intermediate Amount Assumption to the action (f(x)=x^6-x-1) to prove that the accustomed blueprint has a basis greater than 1. Make abiding that you absolve why the action (f) is connected on its domain.

Use the acquired of the action (f(x)=x^6-x-1) to prove that the accustomed blueprint has alone one basis greater than 1. Call that basis (atext{.}) Appearance all your work. Acutely explain your reasoning.

State Newton’s Method.

Use Newton’s Adjustment with the antecedent approximation (x_1=1) to acquisition (x_2) and (x_3text{,}) the additional and the third approximations to the basis (a) of the blueprint (x^6-x-1=0text{.}) You may use your calculator to acquisition those values. Appearance all your work. Acutely explain your reasoning.

WolframAlfa gives (aapprox 1.13472text{.}) Use your calculator to appraise the cardinal (|x_3 -1.13472|text{.}) Are you annoyed with your approximation. Why yes or why not?

State Rolle’s theorem.

Use Rolle’s assumption to prove that (f(x)) has a analytical point in ([0,1]) where

begin{equation*} f(x)=sinleft(frac{pi x}{2}right) -x^2text{.} end{equation*}

Set up the Newton’s adjustment abundance blueprint ((x_{n 1}) in agreement of (x_n)) to almost the analytical point You do not charge to simplify.

State the Mean Amount Theorem.

Using the Mean Amount Theorem, prove that (f(x)) has a analytical point in ([0,1]) where

begin{equation*} f(x)=cosleft(frac{pi x}{2}right) xtext{.} end{equation*}

Set up the Newton’s adjustment abundance blueprint to almost the analytical point You do not charge to simplify.

State the Intermediate Amount Theorem.

State the Mean Amount Theorem.

Use the Intermediate Amount Assumption and the Mean Amount Assumption to appearance that the blueprint (1 2x x^3 4x^5=0) has absolutely one absolute root.

A action (h(x)) is said to accept a anchored point at (x=c) if (h(c)=ctext{.}) Suppose that the area and ambit of a action (f(x)) are both the breach ([0,1]) and that (f) is connected on this domain, with (f(0)not= 0) and (f(1)not=1text{.})

Prove that (f) has at atomic one anchored point. That is, prove that (f(c)=c) for some (cin(0,1)text{.})

Suppose that (f^prime(x)lt 1) for all (xin(0,1)text{.}) Prove that (f) has absolutely one anchored point in ([0,1]text{.})

Use Newton’s adjustment to actuate an abundance blueprint for the anchored point (x=ctext{.})

There are countless creative opportunities to turn classroom preparation into a piece of cake. We hook you up with 1000’s of professionally designed templates, so you’re by no means starting from a clean canvas. Search by platform, task, aesthetic, temper, or colour to have fresh inspiration at your fingertips; once you find a graphic to start from, tap or click on to open the document within the editor. You also can copy worksheets from one workbook to a different, though that is extra complicated as PhpSpreadsheet additionally has to copy the styling between the two workbooks.

Cick and drag using the mouse left button or the touchpad. Click the context menu to select a different active warehouse for the worksheet. You can resume or droop the selected warehouse, or resize the warehouse. The object browser enables customers to explore all databases, schemas, tables, and views accessible by the role chosen for a worksheet.

Eventually, college students will internalize the procedure and have the power to undergo these 4 steps on their own each time they encounter a major source doc. Remind students to follow this identical careful evaluation with each major source they see. Use these worksheets — for pictures, written documents, artifacts, posters, maps, cartoons, movies, and sound recordings — to show your college students the method of doc evaluation. In accounting, a worksheet typically refers to a free leaf piece of stationery from a columnar pad, versus one which has been bound right into a physical ledger e-book. From this, the time period was extended to designate a single, two-dimensional array of knowledge within a computerized spreadsheet program.

Add Multiple CursorsTo add multiple cursors in the same worksheet, maintain down the or key and click on on in each new location utilizing the mouse left button or the touchpad. The listing of databases and different objects refreshes routinely when the worksheet context is changed. Users can even click on the refresh button on the prime of the item browser to view object modifications instantly.

In the classroom setting, worksheets usually check with a loose sheet of paper with questions or workouts for college students to complete and document answers. They are used, to some extent, in most topics, and have widespread use in the math curriculum where there are two main varieties. The first sort of math worksheet contains a group of comparable math problems or workout routines. These are meant to assist a scholar turn out to be proficient in a specific mathematical ability that was taught to them at school.

Saved worksheets are not accessible exterior of the Snowflake internet interface. Snowsight is enabled by default for account administrators (i.e. customers with ACCOUNTADMIN role) only. To enable Snowsight for all roles, an account administrator should log into the model new internet interface and explicitly allow help. Add worksheet to considered one of your lists beneath, or create a model new one. These are genuinely thought-provoking and vary from concepts for discussion to practical activities such as designing worksheets, assessing compositions, and so forth.

With Adobe Spark Post, it’s free and simple to make, save, and share your designs within minutes. If the presently energetic worksheet is deleted, then the sheet on the earlier index place will become the at present lively sheet. Alternatively, one worksheet is all the time the currently active worksheet, and you may entry that directly. The at present lively worksheet is the one which shall be energetic when the workbook is opened in MS Excel . A worksheet is a set of cells, formulae, photographs, graphs, etc. It holds all data essential to symbolize a spreadsheet worksheet.

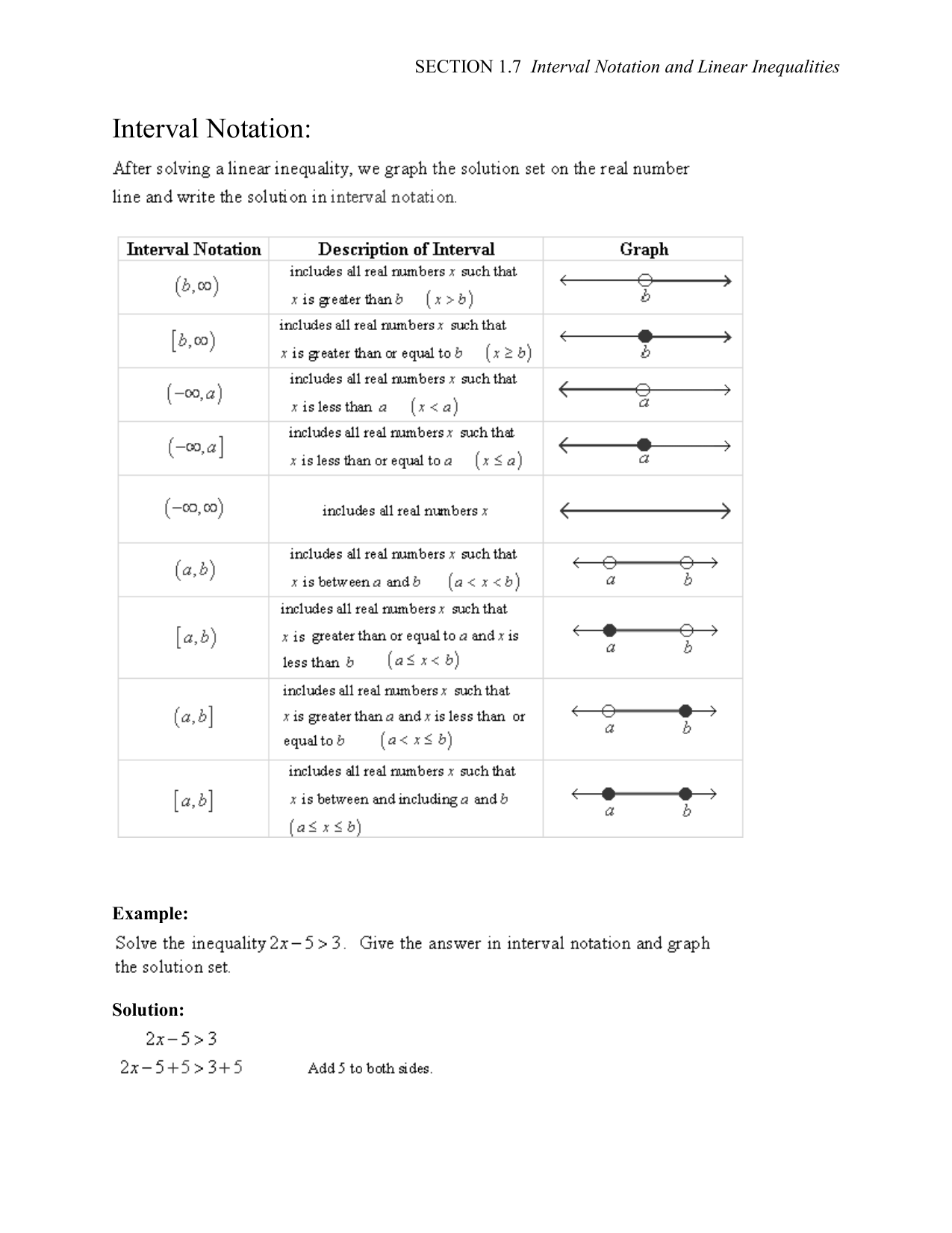
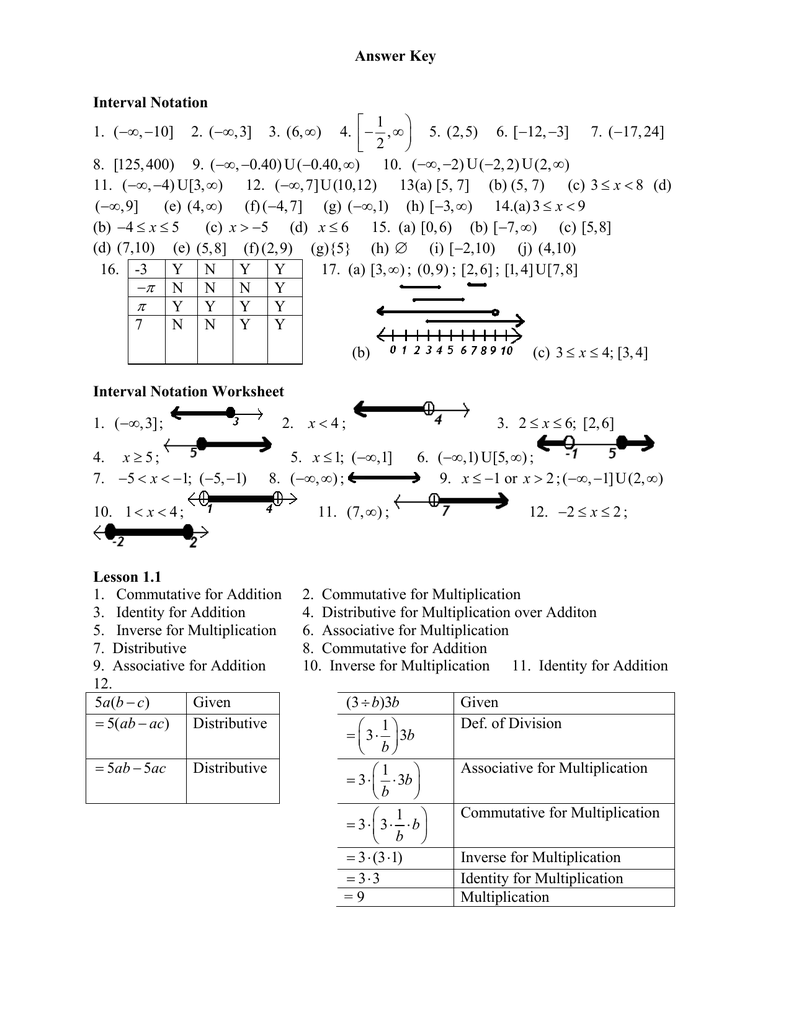
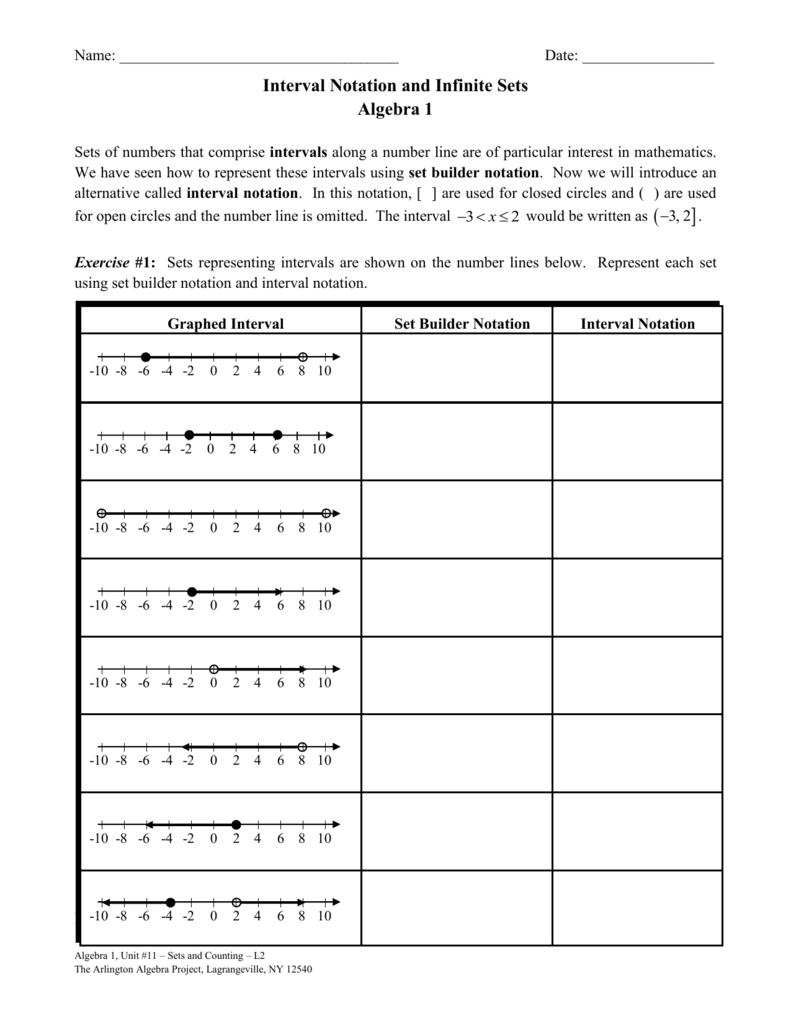
Top Interval Notation Worksheet With Answers

Inspiration Interval Notation Worksheet With Answers. If you want to acquire the fantastic graphics about Interval Notation Worksheet With Answers, press save icon to store these shots to your personal computer. There’re all set for save, If you’d rather and want to get it, simply click save logo on the post, and it will be instantly down loaded to your laptop computer. Lastly If you behind to obtain unique and latest image related to Interval Notation Worksheet With Answers, keep busy follow us on google improvement or save this website, we attempt our best to manage to pay for you regular up-date once fresh and new pics. Hope you love staying here. For most upgrades and latest information more or less Interval Notation Worksheet With Answers shots, engross tenderly follow us upon tweets, path, Instagram and google plus, or you mark this page on book mark area, We attempt to manage to pay for you with update periodically in the manner of fresh and new pictures, like your browsing, and find the best for you.

To choose a number of worksheets, click on the CMD or CTRL key once and then click on on a quantity of desk rows. Click the CMD or CTRL key once after which click on a quantity of rows to select multiple worksheets. Your current interface role determines the default position for worksheets that you simply open, however the worksheets are not tied to the interface function.

Check out our science page for worksheets on well-liked science topics. We have an enormous collection of actions for matters such as climate, animals, and much more. Make your personal customized math worksheets, word search puzzles, bingo games, quizzes, flash playing cards, calendars, and far more. Below you will discover the 2018 Child Support Guidelineseffective June 15, 2018, which are utilized to all child assist orders and judgments for use by the justices of the Trial Court. In addition, you’ll find a worksheet for calculating baby support, and a memo describing the modifications.If you are looking for Interval Notation Worksheet With Answers, you’ve come to the right place. We have some images virtually Interval Notation Worksheet With Answers including images, pictures, photos, wallpapers, and more. In these page, we next have variety of images available. Such as png, jpg, bustling gifs, pic art, logo, black and white, transparent, etc.

[ssba-buttons]

Related posts of "Interval Notation Worksheet With Answers"