Solve Quadratics By Factoring Worksheet. This is a handout with Practice Questions associated to Solving Quadratic Equations by Factoring, and it also accommodates a take a look at with similar questions. Now we now have to divide the 2 factors 4 and -3 by the coefficient of x2, that’s three. Kids will be able to simply review and follow their math abilities. By 1545 Gerolamo Cardano compiled the works associated to the quadratic equations.
To have the power to remedy quadratic equation . To have the power to use the discriminant to search out the number and nature of solutions.

The coefficients may be read off directly. Thus the solutions within the diagram are −AX1/SA and −AX2/SA. Find the square root using a desk of squares.
Remedy A Quadratic Equation By Factoring
Easily examine their work with the reply sheets. Math-Aids Factor List Prime Factorization Worksheet. I can add, subtract and multiply polynomial expressions Factoring Quadratic Expressions 1.

Quadratic Equation Worksheets. Help college students power by way of quadratic equations with this compilation of worksheets dynamically ready to cater to the needs of excessive school students.
Planet Zoo Graphics Settings
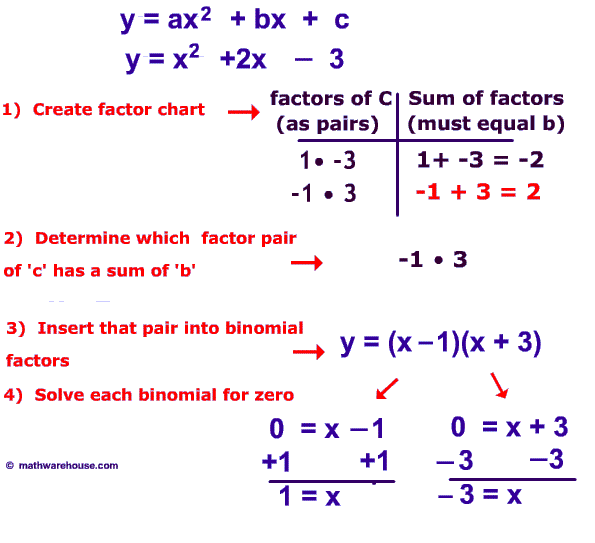
Factoring quadratic equations consists of rewriting the quadratic equation to form a product of its elements. Factoring may be thought of as the reverse means of the multiplication distribution. Here, we will learn about two circumstances of factoring quadratic equations..
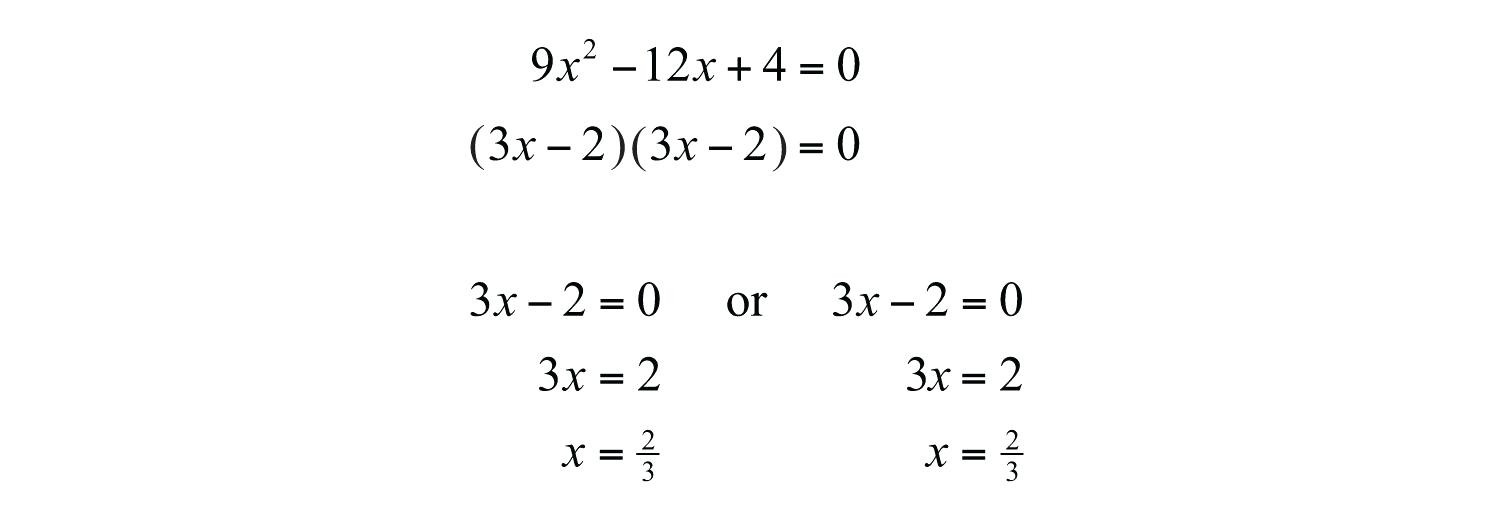
10 given the graph, write the inequality.This product is included within the Graphing Inequalities BundleWorksheets are copyright material and are meant to be used in. X 1 RMva 3dQeI 7wIi it hS iI 3nUfPiQn0iMt1eC APorMem-GA6l yg3e mbor ca9. J Worksheet by Kuta Software LLC Kuta Software – Infinite Pre-Algebra Name_____ Solving One-Step Inequalities by Multiplying/Dividing Date_____ Period____ Solve every.
Apply Solutions
E.g., a( x + b/2a)2 +c – a(b/2a)2. Various strategies are utilized in factorising quadratics, which mainly include splitting the middle term and the quadratic formula. Factoring quadratic worksheets by Cuemath covers each of them.

Predict if the roots are equal or unequal and in addition if they’re actual or complex. A quadratic function is constant.
Algebra And Pre
Abū Kāmil Shujā ibn Aslam in particular was the first to simply accept irrational numbers as options to quadratic equations or as coefficients in an equation. The ninth century Indian mathematician Sridhara wrote down guidelines for fixing quadratic equations. Walk your students through this assortment of pdf worksheets!

Now we’ve to divide the 2 components 4 and -3 by the coefficient of x2, that is 2. Now we now have to divide the two factors four and -3 by the coefficient of x2, that’s three.
However, in some unspecified time in the future the quadratic method begins to lose accuracy because of round off error, while the approximate method continues to enhance. Consequently, the distinction between the strategies begins to increase as the quadratic method turns into worse and worse. The other answer of the identical equation by means of the related radii gives the distance between the circumscribed circle’s middle and the middle of the excircle of an ex-tangential quadrilateral.

But, what if there was a binomial as an alternative of a single time period outdoors of the. This is a graphing inequalities worksheet.10 draw the graph for given inequality.
Completing The Sq Worksheets
Now add 32to both sides to finish the square on the left side of the equation. Critical factors of a cubic function and inflection factors of a quartic operate are found by fixing a quadratic equation.

This is likely certainly one of the fundamental ideas one should be thorough with, to proceed additional in arithmetic. In order to carry out well in matters of algebra, factoring quadratics is doubtless certainly one of the most major concepts one must be taught.
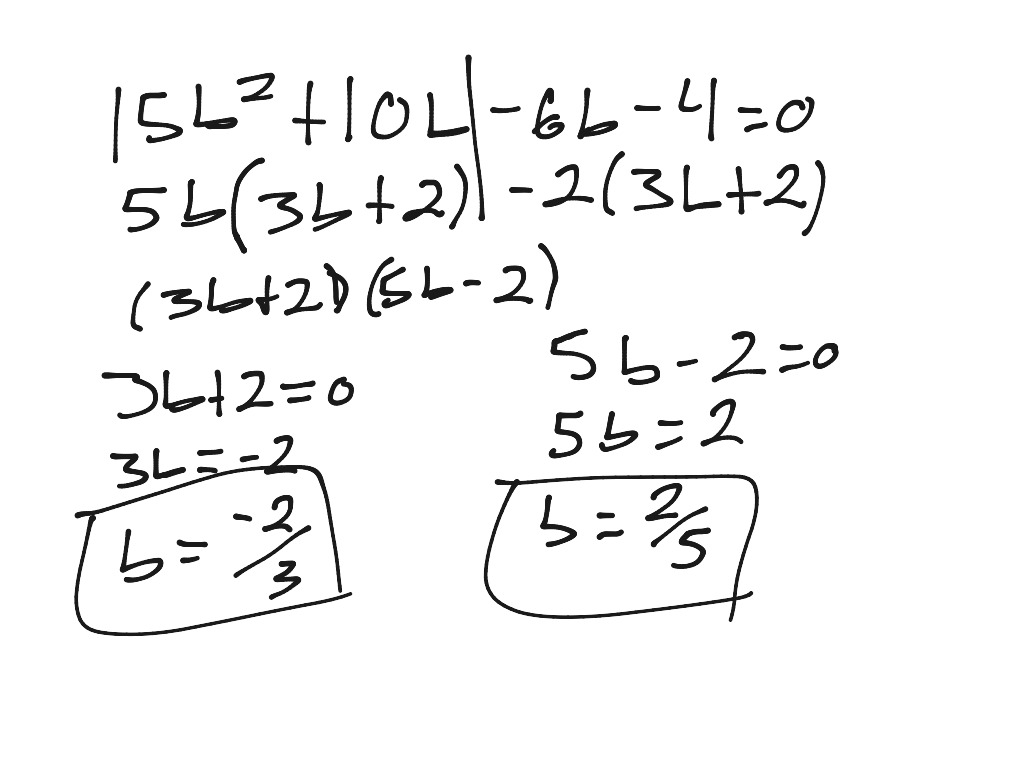
This “completes the sq.”, changing the left facet into an ideal square. Demonstrates tips on how to remedy more difficult issues. Keep all x associated phrases on one aspect.

The options of two roots of quadratic equation with actual or complex coefficients may or will not be distinct, and so they might or will not be actual and the roots can be derived from the method. Progress to the subsequent stage of difficulty by fixing the sophisticated quadratic equations here! Because they’ve an expression rather than the unknown, these equations are known as disguised quadratic equations.
Complete the square of the given quadratic equation and solve for the roots. Level up by working with equations involving radical, fractional, integer, and decimal coefficients.
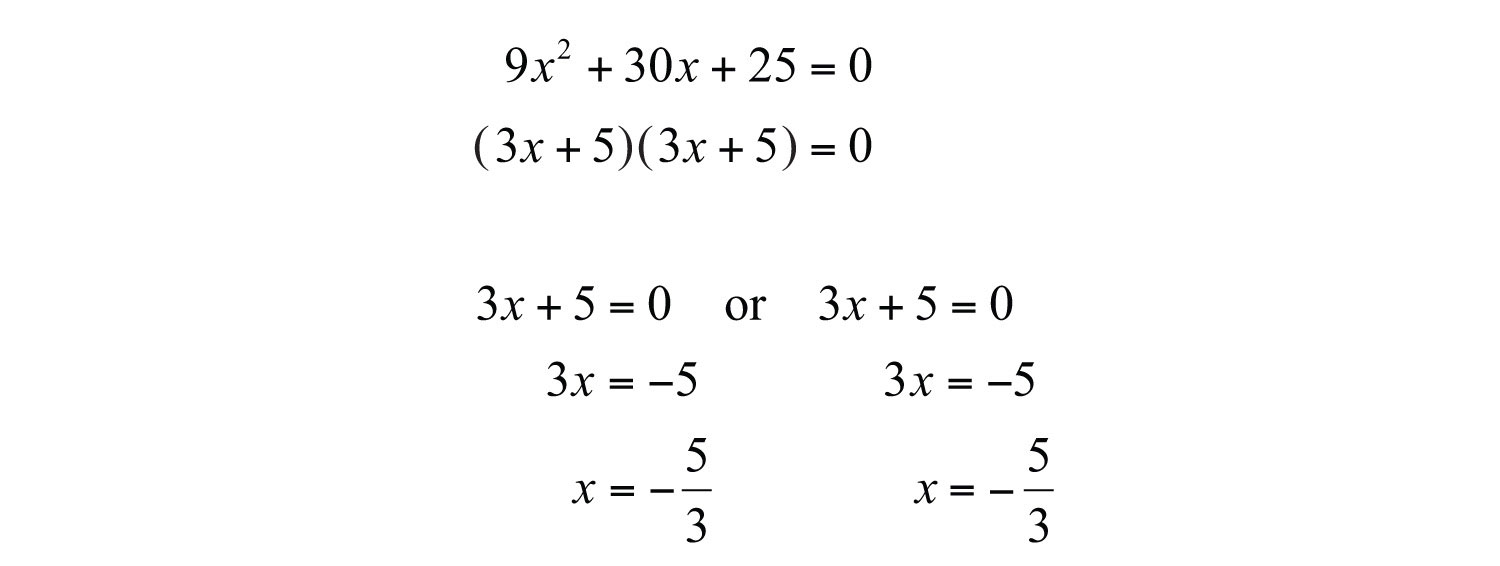
Bundle of five worksheets on solving quadratic equations by using square roots, factoring, and quadratic formulation. Also features a worksheet on utilizing the discriminate of the quadratic formulation to find out the number of options.

If it’s not, divide each term by that value to create a leading coefficient of 1. Learn tips on how to solve a quadratic-linear system using substitution.
Acquaint them with discovering the sum and product of the roots of a given quadratic equation. Equip them to utilize this sum and product to kind the quadratic equation and determine the missing coefficients or constant in it.
Quadratic Equation Solver to find the 2 unknown roots of equation from the enter values a, b and c. Decompose the fixed time period +65 into two elements such that the product of the two elements is equal to +65 and the addition of two factors is equal to the coefficient of x, that’s -18. Decompose the constant time period -7 into two factors such that the product of the 2 elements is the identical as -7 and the addition of two components is the same as the coefficient of x, that is -6.

I can factor when a isn’t equal to 1. I can issue excellent sq. trinomials. I can factor utilizing distinction of squares.

With a purely geometric method Pythagoras and Euclid created a general procedure to find options of the quadratic equation. In his work Arithmetica, the Greek mathematician Diophantus solved the quadratic equation, however giving just one root, even when both roots have been constructive. Keep highschool students au fait with the appliance of square root property in fixing pure quadratic equations, with this assemblage of printable worksheets.

To have the flexibility to sketch the graph of quadratic capabilities. To be capable of find and label key options of parabolas with their coordinates. To be succesful of decide the rule of a parabola from given info.

It is the method by which you may find a way to rewrite the quadratic equation. E.g., ax2 + bx + c into a(x – h)2+k. To graph the parabola, because of a(x – h)2+k, you’ll know the vertex is at .
Characteristics Of Quadratic Functions Worksheet Printable worksheets may be saved as .pdf recordsdata that are opened in your browser with Adobe Acrobat Reader or different PDF reader. Most laptop methods already have this put in but if not, you possibly can acquire it here at no cost. For each quadratic function, find the axis of symmetry of its graph.

Now we have to divide the two components four and -9 by the coefficient of x2, that’s 3. It is possible to make use of factorisation to let you sketch a quadratic graph. As students are finishing, have some college students write the work for each drawback on the board after which discuss the problems as a category.

A quadratic equation must have a squared term as its highest energy. Students can use math worksheets to master a math ability through follow, in a examine group or for peer tutoring.

This situation arises commonly in amplifier design, the place broadly separated roots are desired to make sure a stable operation . Of the discriminant, that’s when the 2 roots are very close. This can lead to lack of up to half of correct significant figures in the roots.

In the quadratic equation above, the coefficient of x2is 1. In the given quadratic equation, the coefficient of x2is not 1. In the given quadratic equation, the coefficient of x2 is 1.
[ssba-buttons]